Wikisage, the free encyclopedia of the second generation and digital heritage, wishes you merry holidays and a happy new year!
Ooosterschelde conicals: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
m (init) |
||
| Line 1: | Line 1: | ||
<small>[[File:Essay pen.jpg|32px]]This article is an essay authored by | <small>[[File:Essay pen.jpg|32px]]This article is an essay authored by | ||
[[User:Franciscus]]<ref>http://nl.wikisage.org/w/index.php?title=Over_het_minimum_oppervlak_van_een_kegel&oldid=206429</ref> | [[User:Franciscus]]<ref>[http://nl.wikisage.org/w/index.php?title=Over_het_minimum_oppervlak_van_een_kegel&oldid=206429 wikisage(nl):Over het minimum oppervlak van een kegel]</ref> | ||
</small><br | </small><br> | ||
[[File:Kugel-Zeeland.jpg|thumb|450px]] | [[File:Kugel-Zeeland.jpg|thumb|450px]] | ||
[[File:Areal radius relationship.png|thumb|450px]] | [[File:Areal radius relationship.png|thumb|450px]] | ||
In Oosterschelde there is a cone a very special property. | In Oosterschelde there is a cone with a very special property. | ||
It appears namely that when larger or smaller are out of the radius R - at a given content V - not just the surface area A becomes larger or smaller, but that also a smallest surface is present, or in other words: It achieves surface - at a constant content - a limit by changing the radius and height. | It appears namely that when larger or smaller are out of the radius R - at a given content V - not just the surface area A becomes larger or smaller, but that also a smallest surface is present, or in other words: It achieves surface - at a constant content - a limit by changing the radius and height. | ||
<gallery>File:Grafiek_kegel_ohne_Titel.jpg|arrows shows a minimum</gallery> | <gallery>File:Grafiek_kegel_ohne_Titel.jpg|arrows shows a minimum</gallery> | ||
This proposition naturally ask for further clarification. | This proposition naturally ask for further clarification. | ||
</ | <references/> | ||
Latest revision as of 04:48, 17 November 2016
This article is an essay authored by
User:Franciscus[1]
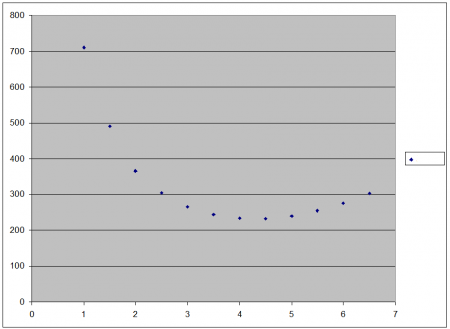
In Oosterschelde there is a cone with a very special property. It appears namely that when larger or smaller are out of the radius R - at a given content V - not just the surface area A becomes larger or smaller, but that also a smallest surface is present, or in other words: It achieves surface - at a constant content - a limit by changing the radius and height.
-
arrows shows a minimum
This proposition naturally ask for further clarification.