Essay:Kunst en Wiskunde I

This article is an essay authored by User:Franciscus

The top row contains 3 triangular bars. Each row increases the number of rods and increases the number of rods. The last row contains 12 twelve-angled bars

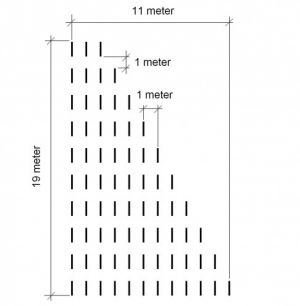
The work on the image -an impressive floor sculpture- was made by the American Walter De Maria (1935-2013) in 1984 on behalf of the Museum Boymans -Van Beuningen in Rotterdam. The name that the artist gives to this work is: A Computer Which Will Solve Every Problem in the World. The work consists of 75 polished stainless steel rods, laid down in rows on the floor, each of which is exactly one meter long. At a first exploration, you immediately notice that the clear and even construction of the floor sculpture gives a new image at each change of viewpoint. In the arrangement on the floor there appears to be strict rules that only last after searching and thinking. It turns out that - starting with a row of three triangular rods - the sculpture ends in a row of twelve - almost round - twelve-angled rods. All rows of bars thus appear to be made up of continually increasing regular polygons 1). Thus, in this arrangement there are ten rows in a corresponding pattern, thus increasing the number of rods as the rows move, so that more and more the shape of a circle is approached. The length of a meter is present throughout the arrangement: also the parallel rows are located at a distance of one meter apart.